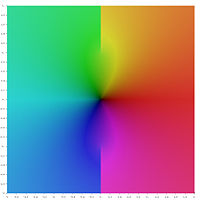
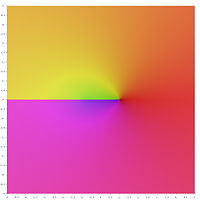
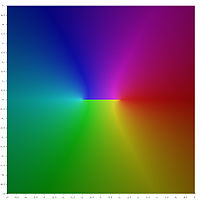
Função hiperbólica inversa

Na matemática, a função hiperbólica inversa fornece um ângulo hiperbólico correspondente a um determinado valor da função hiperbólica. A magnitude do ângulo hiperbólico é equivalente à área do setor hiperbólico da hipérbole unitária xy = 1, ou o dobro da área correspondente ao setor da unidade x² − y² = 1, assim como um ângulo circular é o dobro da área do setor circular de um círculo unitário.^[1]

Quanto à nomenclatura, as abreviaturas preferenciais são arsinh, arcosh e assim por diante, sendo estas representantes das funções trigonométricas inversas. Em outros campos, tal como a ciência da computação, a abreviação é feita pelo prefixo asinh e ainda pode ser válido as notações sinh⁻¹(x), cosh⁻¹(x), entre outras.^[2]

Representação logarítmica

Arco seno hiperbólico

${\displaystyle \operatorname {arsinh} x=\ln(x+{\sqrt {x^{2}+1}})}$

O domínio é o conjunto de números reais.

Demonstração:

${\displaystyle x={\frac {e^{y}-e^{-y}}{2}}\Longleftrightarrow 2xe^{y}=(e^{y})^{2}-1}$

Se ${\displaystyle z=e^{y}\ ,\ y=\ln(z)}$ então ${\displaystyle 2xz=z^{2}-1\Longleftrightarrow z^{2}-2xz-1=0}$

${\displaystyle z_{1,2}={\frac {2x\pm {\sqrt {4x^{2}+4}}}{2}}\ =x\pm {\sqrt {x^{2}+1}}\Longleftrightarrow y_{1,2}=\ln(x\pm {\sqrt {x^{2}+1}})}$

Como ${\displaystyle x-{\sqrt {x^{2}+1}}<0}$, a única solução será ${\displaystyle \ln(x+{\sqrt {x^{2}+1}})}$.

Arco cosseno hiperbólico

${\displaystyle \operatorname {arcosh} x=\ln(x+{\sqrt {x^{2}-1}})}$

O domínio é o intervalo fechado [1, +∞ ).

Demonstração:

${\displaystyle x={\frac {e^{y}+e^{-y}}{2}}\ <==>\ 2xe^{y}=(e^{y})^{2}+1}$

Se ${\displaystyle z=e^{y}\ ,\ y=\ln(z)}$ então ${\displaystyle 2xz=z^{2}+1\ <==>\ z^{2}-2xz+1=0\ <==>\ }$

${\displaystyle z_{1,2}={\frac {2x\pm {\sqrt {4x^{2}-4}}}{2}}\ =x\pm {\sqrt {x^{2}-1}}\ <==>\ y_{1,2}=\ln(x\pm {\sqrt {x^{2}-1}})}$

Como ${\displaystyle x-{\sqrt {x^{2}-1}}={\frac {1}{x+{\sqrt {x^{2}-1}}}}}$ a solução será ${\displaystyle \pm \ln(x+{\sqrt {x^{2}-1}})}$. Após uniformização, temos ${\displaystyle \ln(x+{\sqrt {x^{2}-1}})}$

Arco tangente hiperbólica

${\displaystyle \operatorname {artanh} x={\frac {1}{2}}\ln \left({\frac {1+x}{1-x}}\right)}$

O domínio é o intervalo aberto (−1, 1).

Arco cotangente hiperbólica

${\displaystyle \operatorname {arcoth} x={\frac {1}{2}}\ln \left({\frac {x+1}{x-1}}\right)}$

O domínio é a união dos intervalos (−∞, −1) e (1, +∞).

Arco cossecante hiperbólica

${\displaystyle \operatorname {arcsch} x=\ln \left({\frac {1}{x}}+{\sqrt {{\frac {1}{x^{2}}}+1}}\right)=\ln \left({\frac {1+{\sqrt {1+x^{2}}}}{x}}\right)}$

O domínio é o conjunto dos números reais excluindo o 0.

Arco secante hiperbólica

${\displaystyle \operatorname {arsech} x=\ln \left({\frac {1}{x}}+{\sqrt {{\frac {1}{x^{2}}}-1}}\right)=\ln \left({\frac {1+{\sqrt {1-x^{2}}}}{x}}\right)}$

O domínio é o intervalo semiaberto (0, 1].

Note que devemos considerar o valor principal das raízes quadradas e da função logarítmica citadas acima. No caso de argumentos reais (z = x, onde x é real), algumas simplificações podem ser feitas, como por exemplo, ${\displaystyle {\sqrt {x+1}}{\sqrt {x-1}}={\sqrt {x^{2}-1}}}$ e ${\displaystyle \ln \left({1+x}\right)-\ln \left({1-x}\right)=\ln \left({\tfrac {1+x}{1-x}}\right)}$ .

Fórmulas aditivas

${\displaystyle \operatorname {arsinh} \;u\pm \operatorname {arsinh} \;v=\operatorname {arsinh} \left(u{\sqrt {1+v^{2}}}\pm v{\sqrt {1+u^{2}}}\right)}$

${\displaystyle \operatorname {arcosh} \;u\pm \operatorname {arcosh} \;v=\operatorname {arcosh} \left(uv\pm {\sqrt {(u^{2}-1)(v^{2}-1)}}\right)}$

${\displaystyle \operatorname {artanh} \;u\pm \operatorname {artanh} \;v=\operatorname {artanh} \left({\frac {u\pm v}{1\pm uv}}\right)}$

${\displaystyle {\begin{aligned}\operatorname {arsinh} \;u+\operatorname {arcosh} \;v&=\operatorname {arsinh} \left(uv+{\sqrt {(1+u^{2})(v^{2}-1)}}\right)\\&=\operatorname {arcosh} \left(v{\sqrt {1+u^{2}}}+u{\sqrt {v^{2}-1}}\right)\end{aligned}}}$

Outras identidades

${\displaystyle {\begin{aligned}2\operatorname {arcosh} x&=\operatorname {arcosh} (2x^{2}-1)&\quad {\hbox{ para }}x\geq 1\\4\operatorname {arcosh} x&=\operatorname {arcosh} (8x^{4}-8x^{2}+1)&\quad {\hbox{ para }}x\geq 1\\2\operatorname {arsinh} x&=\operatorname {arcosh} (2x^{2}+1)&\quad {\hbox{ para }}x\geq 0\\4\operatorname {arsinh} x&=\operatorname {arcosh} (8x^{4}+8x^{2}+1)&\quad {\hbox{ para }}x\geq 0\end{aligned}}}$

${\displaystyle \ln(x)=\operatorname {arcosh} \left({\frac {x^{2}+1}{2x}}\right)=\operatorname {arsinh} \left({\frac {x^{2}-1}{2x}}\right)=\operatorname {artanh} \left({\frac {x^{2}-1}{x^{2}+1}}\right)}$

Derivadas das funções hiperbólicas inversas

Derivada de arco seno hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {arsinh} \,x={\frac {1}{\sqrt {x^{2}+1}}}}$

Derivada de arco cosseno hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {arcosh} \,x={\frac {1}{\sqrt {x^{2}-1}}}}$, se ${\displaystyle 1<x}$

Derivada de arco tangente hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {artanh} \,x={\frac {1}{1-x^{2}}}}$, se ${\displaystyle -1<x<1}$

Derivada de arco cotangente hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {arcoth} \,x={\frac {1}{1-x^{2}}}}$, se ${\displaystyle \left\vert x\right\vert >1}$

Derivada de arco secante hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {arsech} \,x=-{\frac {1}{x{\sqrt {1-x^{2}}}}}}$, se ${\displaystyle 0<x<1}$

Derivada de arco cossecante hiperbólico

${\displaystyle {\frac {d}{dx}}\,\operatorname {arcsch} \,x=-{\frac {1}{|x|{\sqrt {1+x^{2}}}}}}$, se ${\displaystyle x\not =0}$

Expansões em série

Expansão em série de arco seno hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {arsinh} \,x&=x-\left({\frac {1}{2}}\right){\frac {x^{3}}{3}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{5}}{5}}-\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{7}}{7}}\pm \cdots \\&=\sum _{n=0}^{\infty }\left({\frac {(-1)^{n}(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{2n+1}}{2n+1}},\qquad \left|x\right|<1\end{aligned}}}$

Expansão em série de arco cosseno hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {arcosh} \,x&=\ln(2x)-\left(\left({\frac {1}{2}}\right){\frac {x^{-2}}{2}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{-4}}{4}}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{-6}}{6}}+\cdots \right)\\&=\ln(2x)-\sum _{n=1}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{-2n}}{2n}},\qquad x>1\end{aligned}}}$

Expansão em série de arco tangente hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {artanh} \,x&=x+{\frac {x^{3}}{3}}+{\frac {x^{5}}{5}}+{\frac {x^{7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }{\frac {x^{2n+1}}{2n+1}},\qquad \left|x\right|<1\end{aligned}}}$

Expansão em série de arco cossecante hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {arcsch} \,x=\operatorname {arsinh} {\frac {1}{x}}&=x^{-1}-\left({\frac {1}{2}}\right){\frac {x^{-3}}{3}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{-5}}{5}}-\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{-7}}{7}}\pm \cdots \\&=\sum _{n=0}^{\infty }\left({\frac {(-1)^{n}(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{-(2n+1)}}{2n+1}},\qquad \left|x\right|>1\end{aligned}}}$

Expansão em série de arco secante hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {arsech} \,x=\operatorname {arcosh} {\frac {1}{x}}&=\ln {\frac {2}{x}}-\left(\left({\frac {1}{2}}\right){\frac {x^{2}}{2}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right){\frac {x^{4}}{4}}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right){\frac {x^{6}}{6}}+\cdots \right)\\&=\ln {\frac {2}{x}}-\sum _{n=1}^{\infty }\left({\frac {(2n)!}{2^{2n}(n!)^{2}}}\right){\frac {x^{2n}}{2n}},\qquad 0<x\leq 1\end{aligned}}}$

Expansão em série de arco cotangente hiperbólico:

${\displaystyle {\begin{aligned}\operatorname {arcoth} \,x=\operatorname {artanh} {\frac {1}{x}}&=x^{-1}+{\frac {x^{-3}}{3}}+{\frac {x^{-5}}{5}}+{\frac {x^{-7}}{7}}+\cdots \\&=\sum _{n=0}^{\infty }{\frac {x^{-(2n+1)}}{2n+1}},\qquad \left|x\right|>1\end{aligned}}}$

Representação gráfica

${\displaystyle \operatorname {arsinh} (z)}$

${\displaystyle \operatorname {arcosh} (z)}$

${\displaystyle \operatorname {artanh} (z)}$

${\displaystyle \operatorname {arcoth} (z)}$

${\displaystyle \operatorname {arsech} (z)}$

${\displaystyle \operatorname {arcsch} (z)}$

Funções hiperbólicas inversas no plano-z complexo: a cor em cada ponto do plano representa o valor complexo da respectiva função que condiciona tal ponto.

Referências

Ligações externas

• Hazewinkel, Michiel, ed. (2001), «Inverse hyperbolic functions», Enciclopédia de Matemática, ISBN 978-1-55608-010-4 (em inglês), Springer